Page 45 - Chip Scale Review_January February_2023-digital

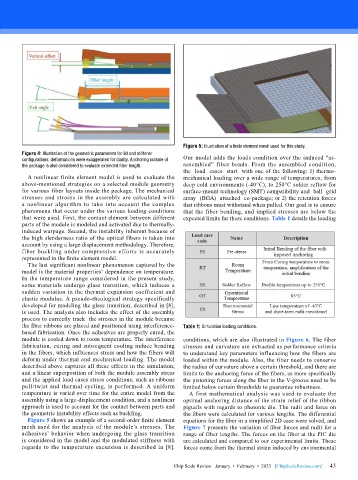
Figure 5: Illustration of a finite element mesh used for this study.
Figure 4: Illustration of the geometric parameters for lid and stiffener
configurations; deformations were exaggerated for clarity. Anchoring outside of Our model adds the loads condition over the induced “as-
the package is also considered to evaluate extended fiber length. assembled” fiber bends. From the assembled condition,
the load cases start with one of the following: 1) thermo-
A nonlinear finite element model is used to evaluate the mechanical loading over a wide range of temperatures, from
above-mentioned strategies on a selected module geometry deep cold environments (-40°C), to 250°C solder reflow for
for various fiber layouts inside the package. The mechanical surface-mount technology (SMT) compatibility and ball grid
stresses and strains in the assembly are calculated with array (BGA) attached co-package; or 2) the retention forces
a nonlinear algorithm to take into account the complex that ribbons must withstand when pulled. Our goal is to ensure
phenomena that occur under the various loading conditions that the fiber bending, and implied stresses are below the
that were used. First, the contact element between different expected limits for these conditions. Table 1 details the loading
parts of the module is modeled and activated due to thermally-
induced warpage. Second, the instability inherent because of
the high slenderness ratio of the optical fibers is taken into
account by using a large displacement methodology. Therefore,
fiber buckling under compressive efforts is accurately
represented in the finite element model.
The last significant nonlinear phenomenon captured by the
model is the material properties’ dependence on temperature.
In the temperature range considered in the present study,
some materials undergo glass transition, which induces a
sudden variation in the thermal expansion coefficient and
elastic modulus. A pseudo-rheological strategy specifically
developed for modeling the glass transition, described in [8],
is used. The analysis also includes the effect of the assembly
process to correctly track the stresses in the module because
the fiber ribbons are placed and positioned using interference- Table 1: Simulation loading conditions.
based fabrication. Once the adhesives are properly cured, the
module is cooled down to room temperature. The interference conditions, which are also illustrated in Figure 6. The fiber
fabrication, curing and subsequent cooling induce bending stresses and curvature are extracted as performance criteria
in the fibers, which influences stress and how the fibers will to understand key parameters influencing how the fibers are
deform under thermal and mechanical loading. The model loaded within the module. Also, the fiber needs to conserve
described above captures all these effects in the simulation, the radius of curvature above a certain threshold, and there are
and a linear superposition of both the module assembly stress limits to the anchoring force of the fibers, as more specifically
and the applied load cases stress conditions, such as ribbons the pistoning forces along the fiber in the V-groove need to be
pull/twist and thermal cycling, is performed. A uniform limited below certain thresholds to guarantee robustness.
temperature is varied over time for the entire model from the A first mathematical analysis was used to evaluate the
assembly using a large-displacement condition, and a nonlinear optimal anchoring distance of the strain relief of the ribbon
approach is used to account for the contact between parts and pigtails with regards to photonic die. The radii and force on
the geometric instability effects such as buckling. the fibers were calculated for various lengths. The differential
Figure 5 shows an example of a second-order finite element equations for the fiber in a simplified 2D case were solved, and
mesh used for the analysis of the module’s stresses. The Figure 7 presents the variation of fiber forces and radii for a
adhesives’ behavior when undergoing the glass transition range of fiber lengths. The forces on the fiber at the PIC die
is considered in the model and the modulated stiffness with are calculated and compared to our experimental limits. These
regards to the temperature excursion is described in [8]. forces come from the thermal strain induced by environmental

43
Chip Scale Review January • February • 2023 [ChipScaleReview.com] 43